Write a Nuclear Equation for the Alpha Decay of 241 95 Am
25: Nuclear Chemistry
-
- Last updated
- Save as PDF
- Page ID
- 8297
These are homework exercises to accompany the Textmap created for "General Chemistry: Principles and Modern Applications " by Petrucci et al. Complementary General Chemistry question banks can be found for other Textmaps and can be accessed here. In addition to these publicly available questions, access to private problems bank for use in exams and homework is available to faculty only on an individual basis; please contact Delmar Larsen for an account with access permission.
Q25.1A
What is/are the product(s) of this nuclear reaction?
- \(\ce{^{26}_{13}Al}\) goes through neutron bombardment
- \(\ce{^{11}_6C}\) goes through positron emission
S25.1A
- \(\mathrm{\ce{^{26}_{13}Al} + \ce{^0_{-1}e} \rightarrow {^{26}_{12}Mg}}\)
- \(\mathrm{\ce{^{11}_6C} \rightarrow \ce{^{11}_{5}B} + {^0_{1}e}}\)
Q25.1B
Transcribe the following descriptions of nuclear reactions into equation form.
- \(\ce{^{238}U}\) decays by alpha particle emission.
- \(\ce{^{234}Th}\) decays by beta particle emission.
S25.1B
- Note that the atomic numbers add to 92 and that the atomic masses add to 238 on both sides of the equation. \[\ce{^{238}_{92}U \rightarrow ^{234}_{90}Th + ^4_2He^{2+}}\]
- Note that the atomic numbers add to 90 and that the a mass numbers add to 234 on both sides of the equation. \[\ce{^{234}_{90}Th \rightarrow \, ^{234}_{91}Pa +\, ^0_{-1}\beta}\]
Q25.1C
- What nuclide results from alpha decay of \(\ce{^{232}Pa}\)?
- What nuclide results from β- decay of \(\ce{^{243}Am}\)?
S25.1C
- \(\ce{^{243}Am \rightarrow \, ^{243}Cm + _{-1}\beta}\)
- \(\ce{^{232}Pa \rightarrow \, ^{228}Ac + ^4He}\)
Q25.1D
Show the nuclear reaction of bottom process (hint: what nucleus is obtained)
- \(\ce{^{201}_{81}Tl}\) decay by \(\alpha\) emission.
- \(\ce{^{238}_{93}Np}\) decay by \(\beta^-\) emission.
S25.1D
- A \(\alpha\) emission causes alpha particles emanating from radioactive nuclei are identical to the nuclei of helium-4 atoms has \(\ce{^{4}_{2}He^2+}\). Which create reactions \(\ce{^{201}_{81}Tl \rightarrow ^{197}_{79}Au + ^{4}_{2}He^2+}\).
- \(\ce{^{238}_{93}Np}\) has a mass number of 238 and atomic number of 93. A positron has a mass number of 0 and it is effective atomic number of +1. Emission of the positron has effect of transforming a proton into a neutron. The parent nuclide must be \(\ce{^{238}_{93}Np \rightarrow ^{238}_{92}U + ^0_{+1}\beta}\).
Q25.1E
Write the Equation for each process
- \(\ce{^{231}_{91}Pa}\) alpha decay
- \(\ce{^{249}_{96}Cm}\) beta emission
S25.1E
- \(\mathrm{\ce{^{231}_{91}Pa} \rightarrow \ce{^{227}_{89}Ac} + {^4_2He}}\)
- \(\mathrm{\ce{^{249}_{96}Cm} \rightarrow \ce{^{249}_{97}Bk} + \ce{^0_{-1}e}}\)
Q25.1F
What nucleus is obtained in each process
- \( \ce{ ^{234}_{94}Pu} \) after decaying by \(\alpha\) emission
- \( \ce{^{248}_{97}Bk}\) after decaying by \(\beta^-\) emission
S25.1F
- Decay by \(\alpha\) emission means losing a \( \ce{^4_2He}\) particle from the atom. So the reaction is asking
\[ \ce{ ^{234}_{94}Pu \rightarrow ^{230}_{92}X + ^4_2He}\]
Given that the new nucleus has an atomic number 92, the nucleus obtained is Uranium-230 or \(\ce{^{230}_{94}U}\)
- Decay by \(\beta^-\) emission means an increase in one atomic number with no change in mass number, so the reaction is asking
\[ \ce{ ^{248}_{97}Bk \rightarrow ^{248}_{98}X + ^0_{-1}\beta }\]
The new atomic number will be the element with number 98, due to the gain is atomic number from losing a beta particle. This nucleus obtained is Californium-248 or \( \ce{^{248}_{98}Cf}\)
To review nuclear reactions, please visit the page "Decay Pathways".
Q25.2A
Write the following nuclear reactions:
- bombardment of \(\ce{^9Be}\) with protons to produce \(\ce{^{10}B}\) and \(\gamma\)(gamma) rays
- bombardment of \(\ce{^7Li}\) with \(\ce{^2H}\) to produce \(\ce{^8Be}\)
- bombardment of \(\ce{^{12}C}\) with neutrons to produce \(\ce{^{12}B}\)
S25.2A
- \(\ce{^9Be + ^1H \rightarrow ^{10}B + \gamma}\)
- \(\ce{^7Li + ^2H \rightarrow ^8Be + ^1n}\)
- \(\ce{^{12}C + ^1n \rightarrow ^{12}B + ^1H}\)
Q25.3A
Write the nuclear equation for the formation an isotope of element 110 with mass number 271 by the bombardment of bismuth-209 by cobalt-63 nuclei, then five alpha particle emissions.
S25.3A
\(\ce{^{209}Bi + ^{63}Co \rightarrow ^{271}Ds + ^1n}\)
\(\ce{^{271}Ds \rightarrow ^{54}He + ^{251}Fm}\)
Q25.4A
The disintegration rate for a sample containing \(\ce{^{60}Co}\) is 6635 dis h-1. The half-life of \(\ce{^{60}Co}\) is 5.2 years. How many atoms of \(\ce{^{60}Co}\) are in the sample?
S25.4A
Determine \(\lambda\)
\(\mathrm{\lambda = \left(\dfrac{0.693}{5.2\,y}\right) \times \left(\dfrac{1\, y}{365.35\, d}\right) \times \left(\dfrac{1\, d}{24\, h}\right) = 1.52 \times 10^{-5}\,h^{-1}}\)
Determine number of atoms
\(\mathrm{N = \dfrac{rate}{\lambda} = \dfrac{6635\, atoms/h}{1.52 \times 10^{-5}\,h^{-1}} = 4.37 \times 10^8}\)
Q25.4B
Scientists that worked on the Manhattan project made many discoveries about the nature of radioactivity. Plutonium is formed by the bombardment of Uranium-238 with neutrons, which leads to the successive emission of two beta particles in two distinct steps. What are the nuclear equations for this three-step process?
S25.4B
We know that the starting parent nuclide is Uranium-238 and that the final daughter nuclide (after a three step reaction) is Plutonium. So, the solution entails writing the equations in three distinct steps.
- \(\mathrm{\ce{^{238}_{92}U} + {^1_0n} \rightarrow \ce{^{239}_{92}U}}\)
\(\mathrm{\textrm{A: } 238 + 1 = 239}\)
\(\mathrm{\textrm{Z: }92 + 0 = 92}\)
- \(\mathrm{\ce{^{239}_{92}U} \rightarrow \ce{^{239}_{93}Np} + \ce{^0_{-1}\beta}}\)
\(\mathrm{\textrm{A: }239 = 239 + 0}\)
\(\mathrm{\textrm{Z: } 92 = 93 +(-1)}\)
- \(\mathrm{\ce{^{239}_{93}Np} \rightarrow \ce{^{239}_{94}Pu} + \ce{^0_{-1}\beta}}\)
\(\mathrm{\textrm{A: }239 = 239 + 0}\)
\(\mathrm{\textrm{Z: }93 = 94 +(-1)}\)
Q25.5A
A sample with \(\ce{^{124}Sb}\) has an activity 100 times the detectable limit. How long would an experiment run before the radioactivity could no longer be detected?
S25.5A
\(\mathrm{Half\: life\: of\: ^{124}Sb = 60.20\: days}\)
\(\mathrm{\lambda = \dfrac{0.693}{60.20\, d} = 0.0115\, d^{-1}}\)
\(\mathrm{\ln\left(\dfrac{1}{100}\right) = -0.0115\, d^{-1} (t)}\)
\(\mathrm{t= 400\: days}\)
Q25.10A
Complete the following nuclear reactions.
- \(\ce{^{39}_{19}K + \color{green}{X} \rightarrow ^{40}_{19}K + ^1_1H }\)
- \(\ce{^{56}_{26}Fe + ^1_0n \rightarrow ^{53}_{24}Cr + \color{red}{X} }\)
- \(\ce{ \color{red}{X} + ^2_1H \rightarrow ^{231}_{91}Pu + ^0_{-1}\beta} \)
- \(\ce{ ^{232}_{90}Th + \color{red}{X} \rightarrow ^{240}_{96}Cm + 5^1_0n} \)
- \(\ce{^{227}_{89}Ac + \color{red}{X} \rightarrow ^{235}_{96}Es + 6 ^1_0n} \)
S25.10A
- \(\ce{^{39}_{19}K + ^2_1H \rightarrow ^{40}_{19}K + ^1_1H }\)
- \(\ce{^{56}_{26}Fe + ^1_0n \rightarrow ^53_{24}Cr + ^4_2He }\)
- \(\ce{^{229}_{89}Ac + ^2_1H \rightarrow ^{231}_{91}Pu + ^0_{-1}\beta }\)
- \(\ce{^{232}_{90}Th + ^{13}_6C \rightarrow ^{240}_{96}Cm + 5^1_0n }\)
- \(\ce{^{227}_{89}Ac + ^{14}_7N \rightarrow ^{235}_{96}Es + 6^1_0n }\)
To review nuclear reactions, please visit the page "Decay Pathways".
Q25.10B
Fill in the blank of these nuclear reactions
- \(\mathrm{\underline{\hspace{20 pt}} + \ce{^{14}_7N} \rightarrow \ce{^{17}_{8}O} + {^1_{1}H}}\)
- \(\mathrm{\ce{^{195}_{79}Au} + \ce{^{1}_0n} \rightarrow \ce{^{192}_{77}Ir} + \underline{\hspace{20 pt}}}\)
- \(\mathrm{\underline{\hspace{20 pt}} + \ce{^{2}_{1}H} \rightarrow \ce{^{225}_{88}Ra} + \ce{^{0}_{-1}\beta}}\)
- \(\mathrm{\ce{^{214}_{85}At} + \underline{\hspace{20 pt}} \rightarrow \ce{^{224}_{92}U} + \ce{4^{1}_{0}n}}\)
- \(\mathrm{\ce{^{235}_{88}Ra} + \underline{\hspace{20 pt}} \rightarrow \ce{^{244}_{93}Cm} + \ce{7^{1}_{0}n}}\)
S25.10B
- \(\ce{^{4}_2He}\)
- \(\ce{^{4}_2He}\)
- \(\ce{^{223}_{86}Rn}\)
- \(\ce{^{14}_{7}N}\)
- \(\ce{^{16}_{8}O}\)
Q25.10C
Complete the following nuclear equations
- \(\mathrm{\ce{^{24}_{12}Mg} + {?} \rightarrow {^{25}_{11}Na} + {^1_1H}}\)
- \(\mathrm{\ce{^{60}_{28}Ni} + {^0_1n} \rightarrow {^{57}_{26}Fe} + {?}}\)
- \(\mathrm{\ce{?} + {^2_1H} \rightarrow \ce{^{243}_{95}Am} + \ce{^0_{-1}\beta}}\)
- \(\mathrm{\ce{^{247}_{97}Bk} + {?} \rightarrow {^{254}_{102}No} + 5^1_0n}\)
- \(\mathrm{\ce{^{239}_{93}Np} + {?} \rightarrow \ce{^{246}_{99}Es} + 6^1_0n}\)
S25.10C
- \(\mathrm{\ce{^{24}_{12}Mg} + {^2_1H} \rightarrow {^{25}_{11}Na} + {^1_1H}}\)
- \(\mathrm{\ce{^{60}_{28}Ni} + {^0_1n} \rightarrow {^{57}_{26}Fe} + {^4_2He}}\)
- \(\mathrm{\ce{^{241}_{93}Np} + {^2_1H} \rightarrow \ce{^{243}_{95}Am} + \ce{^0_{-1}\beta}}\)
- \(\mathrm{\ce{^{247}_{97}Bk} + \ce{^{12}_5B} \rightarrow {^{254}_{102}No} + 5^1_0n}\)
- \(\mathrm{\ce{^{239}_{93}Np} + \ce{^{15}_6C} \rightarrow \ce{^{246}_{99}Es} + 6^1_0n}\)
Q25.10D
Complete the following nuclear equations:
- \(\mathrm{^{37}_{19}K \rightarrow \underline{\hspace{20 pt}} + \ce{^0_{-1}\beta}}\)
- \(\mathrm{\ce{^{239}_{94}Pu} \rightarrow \underline{\hspace{20 pt}} + \ce{^4_2He^2+}}\)
- \(\mathrm{\ce{^{235}_{92}U} + {^1_0n} \rightarrow \underline{\hspace{20 pt}} + 2^1_0n + \ce{^{137}_{52}Te}}\)
- \(\mathrm{\ce{^{241}_{95}Am} + {^4_2He^{2+}} \rightarrow \underline{\hspace{20 pt}} + 2^1_0n}\)
- \(\mathrm{^9_4Be + {^1_1H} \rightarrow \underline{\hspace{20 pt}} + {^4_2He^{2+}}}\)
S25.10D
Nuclear equations of this complexity are a matter of algebraic calculation. The atomic number and mass number of the products must add to the same value (except when relativistic effects are taken into account.) The mass number of an element is denoted '\(\ce{A}\)' and the atomic number is denoted '\(\ce{Z}\)'.
In solving the simple equations for each number, we also determine the isotope of a specific species. The '\(\ce{Z}\)' number (atomic number) tells us where to look on the periodic table and the '\(\ce{A}\)' number is the isotopic number for the missing species from the equation.
- \(\mathrm{^{37}_{19}K \rightarrow {^x_xx} + \ce{^0_{-1}\beta}}\)
\(\mathrm{\textrm{A: } 37 = x + 0 \Rightarrow x = 37}\)
\(\mathrm{\textrm{A: }37 = 37 + 0}\)
\(\mathrm{\textrm{Z: }19 = x +(-1) \Rightarrow x = 20}\)
\(\mathrm{\textrm{Z: }19 = 20 +(-1)}\)
\(\mathrm{^{37}_{19}K \rightarrow \mathbf{^{37}_{20}Ca} + \ce{^0_{-1}\beta}}\)
Potassium, when emitting a Beta particle, gains one proton and thus becomes Calcium.
- \(\mathrm{\ce{^{239}_{94}Pu} \rightarrow {^x_xx} + {^4_2He^{2+}}}\)
\(\mathrm{\textrm{A: } 239 = x + 4 \Rightarrow x = 235}\)
\(\mathrm{\textrm{A: }239 = 235 + 4}\)
\(\mathrm{\textrm{Z: }94 = x + 2 \Rightarrow x = 92}\)
\(\mathrm{\textrm{Z: }94 = 92 + 2}\)
\(\mathrm{\ce{^{239}_{94}Pu} \rightarrow \mathbf{\ce{^{235}_{92}U}} + {^4_2He^{2+}}}\)
Plutonium decays by alpha particle emission to Uranium-235.
- \(\mathrm{\ce{^{235}_{92}U} + {^1_0n} \rightarrow {^x_xx} + 2^1_0n + \ce{^{137}_{52}Te}}\)
\(\mathrm{\textrm{A: } 235 + 1 = x + 2(1) + 13 \Rightarrow x = 97}\)
\(\mathrm{\textrm{A: }235 + 1 = 97 + 2(1) + 13}\)
\(\mathrm{\textrm{Z: }92 + 0 = x + 2(0) + 52 \Rightarrow x = 40}\)
\(\mathrm{\textrm{Z: }92 + 0 = 40 + 2(0) + 52}\)
\(\mathrm{\ce{^{235}_{92}U} + {^1_0n} \rightarrow \mathbf{\ce{^{97}_{40}Zr}} + 2^1_0n + \ce{^{137}_{52}Te}}\)
Uranium-235 is bombarded with neutrons to produce Zirconium-97, 2 neutrons and Tellurium-137. This is an example of a fission reaction.
- \(\mathrm{\ce{^{241}_{95}Am} + {^4_2He^{2+}} \rightarrow {^x_xx} + 2^1_0n}\)
\(\mathrm{\textrm{A: }241 + 4 = x + 2(1) \Rightarrow x = 243}\)
\(\mathrm{\textrm{A: }241 + 4 = 243 + 2(1)}\)
\(\mathrm{\textrm{Z: }95 + 2 = x + 2(0) \Rightarrow x = 97}\)
\(\mathrm{\textrm{Z: }95 + 2 = 97 + 2(0)}\)
\(\mathrm{\ce{^{241}_{95}Am} + {^4_2He^{2+}} \rightarrow \mathbf{\ce{^{243}_{97}Bk}} + 2^1_0n}\)
Americium-241 is bombarded with alpha particles to produce Berkelium-243 and 2 neutrons.
- \(\mathrm{^9_4Be + {^1_1H} \rightarrow {^x_xx} + {^4_2He^{2+}}}\)
\(\mathrm{\textrm{A: }9 + 1 = x + 4 \Rightarrow x = 6}\)
\(\mathrm{\textrm{A: }9 + 1 = 6 + 4 }\)
\(\mathrm{\textrm{Z: }4 + 1 = x + 2 \Rightarrow x = 3}\)
\(\mathrm{\textrm{Z: }4 + 1 = 3 + 2 }\)
\(\mathrm{^9_4Be + {^1_1H} \rightarrow \mathbf{\ce{^6_3Li}} + {^4_2He^{2+}}}\)
Beryllium-9 is bombarded with protons to produce Lithium-6 and an alpha particle.
Q25.10E
Complete the given nuclear reactions below:
- \(\ce{^{24}_{12}Mg + ? \rightarrow ^{27}_{13}Al + ^1_{1}H}\)
- \(\ce{^{56}_{25}Mn + ^1_0n \rightarrow ^{55}_{24}Cr + ?}\)
- \(\ce{? + ^2_1H \rightarrow ^{238}_{91}Pa + ^0_{-1}\beta}\)
- \(\ce{^{237}_{91}Pa + ? \rightarrow ^{250}_{98}Cf + 6^1_{0}n}\)
- \(\ce{^{243}_{95}Am + ? \rightarrow ^{251}_{102}No + 5^1_{0}n}\)
S25.10E
- \(\ce{^{24}_{12}Mg + ^4_2He \rightarrow ^{27}_{13}Al + ^1_{1}H}\)
- \(\ce{^{56}_{25}Mn + ^1_0n \rightarrow ^{55}_{24}Cr + ^2_1H}\)
- \(\ce{^{236}_{91}Pa + ^2_1H \rightarrow ^{238}_{91}Pa + ^0_{-1}\beta}\)
- \(\ce{^{237}_{91}Pa + ^{19}_7N \rightarrow ^{250}_{98}Cf + 6^1_{0}n}\)
- \(\ce{^{243}_{95}Am + ^{13}_7N \rightarrow ^{251}_{102}No + 5^1_{0}n}\)
Q25.11A
Write equations for the following nuclear reactions:
- Bombardment of \(\ce{^{234}Th}\) with \(\alpha \) particles to produce \(\ce{^{238}U}\)
- Bombardment of \(\ce{^{234}Pa}\) with hydrogen atoms to produce \(\ce{^{235}U}\)
- Bombardment of \(\ce{^{238}U}\) with neutrons to produce \(\ce{^{239}U}\) and \(\gamma\) rays and then \(\beta\)-decay of \(\ce{^{239}U}\)
S25.11A
- \(\ce{^{234}_{90}Th + ^4_2He \rightarrow ^{238}_{92}U}\)
- \(\ce{^{234}_{91}Pa + ^1_1H \rightarrow ^{235}_{92}U}\)
- \(\ce{^{238}_{92}U + ^1_0n \rightarrow ^{239}_{92}U + \lambda}\)
- \(\ce{^{239}_{92}U \rightarrow ^{239}_{93}Np + ^0_{-1}\beta}\)
To review nuclear reactions, visit the page "Nuclear Chemistry"
Q25.11B
Show the equations for the following reactions:
- Bombardment of \(\ce{^{235}U}\) with a neutron to produce \(\ce{^{141}Ba}\), \(\ce{^{92}Kr}\), and neutrons
- Bombardment of \(\ce{^{70}Ga}\) with \(\ce{^{2}_{1}H}\) to produce \(\ce{^{72}Ge}\)
- Bombardment of \(\ce{^{121}Sb}\) with alpha particles to produce \(\ce{^{128}Cs}\) and a neutron
S25.11B
- \(\mathrm{{^1_0n} + \ce{^{235}_{92}U} \rightarrow \ce{^{141}_{56}Ba} + {^{92}_{36}Kr} + 3^1_0n}\)
- \(\mathrm{\ce{^{70}_{31}Ga} + {^2_1H} \rightarrow {^{72}_{32}Ge}}\)
- \(\mathrm{\ce{^{121}_{51}Sb} + 2^4_2He \rightarrow \ce{^{128}_{55}Cs} + {^1_0n}}\)
Q25.11C
Complete the equations of the nuclear reactions below
- bombardment of \(\ce{^9Be}\) with protons to produce \(\ce{^{10}Be}\) and γ rays
- bombardment of \(\ce{^{11}B}\) with \(\ce{^2_1H}\) to produce \(\ce{^{12}C}\)
- bombardment of \(\ce{^{16}O}\) with neutrons to produce \(\ce{^{16}N}\)
S25.11C
- \(\mathrm{\ce{^9_4Be} + {^1_1H} \rightarrow \ce{^{10}_5B} + \gamma}\)
- \(\mathrm{\ce{^{11}_5B} + {^2_1H} \rightarrow \ce{^{12}_6C} + {^1_0n}}\)
- \(\mathrm{\ce{^{16}_8O} + {^1_0n} \rightarrow \ce{^{16}_7N} + {^1_1H}}\)
Q25.11D
Write equations for the following nuclear reactions:
- Alpha decay of \(\ce{^{210}Bi}\)
- Beta decay of \(\ce{^{210}Bi}\)
- Fusion of Californium-252 and Boron-10
S25.11D
- This reaction shows the decay of Bismuth-210 into a daughter species and an alpha particle.
\(\mathrm{\ce{^{210}_{83}Bi} \rightarrow \ce{^{206}_{81}Tl} + {^4_2He^{2+}}}\)
\(\mathrm{\textrm{A: } 210 - 4 = 206}\)
\(\mathrm{\textrm{Z: }83 \:(\textrm{the atomic number of Bismuth}) - 2 = 81}\)
- This reaction shows another pathway of decay for Bismuth-210, this time by emission of a beta particle to form a daughter species.
\(\mathrm{\ce{^{210}_{83}Bi} \rightarrow \ce{^{210}_{84}Po} + \ce{^0_{-1}\beta}}\)
\(\mathrm{\textrm{A: }210 - 0 = 210}\)
\(\mathrm{\textrm{Z: }83 -(-1) = 84}\)
- This reaction involves two parent nuclides and results in one daughter nuclide.
\(\mathrm{\ce{^{252}_{98}Cf} + \ce{^{10}_5B} \rightarrow {^{262}_{103}Lr}}\)
\(\mathrm{\textrm{A: } 252 + 10 = 262}\)
\(\mathrm{\textrm{Z: }98 + 5 = 103}\)
Q25.11E
Convert the word in to equations for the below nuclear reactions.
- Bombardment of \(\ce{^7_4Be}\) with protons to produce \(\ce{^8_5B}\) and \(\gamma\) rays
- Bombardment of \(\ce{^{17}_{12}Mg}\) with \(\ce{^{3}_{1}H}\) to produce \(\ce{^{19}_{13}Al}\) and \(\ce{^{1}_{0}n}\)
- Bombardment of \(\ce{^{22}_{15}P}\)with neutrons to produce\(\ce{^{22}_{14}Si}\)
S25.11E
- \(\ce{^7_4Be + ^{1}_1H \rightarrow ^9_5B + \gamma}\)
- \(\ce{^{17}_{12}Mg + ^{3}_{1}H \rightarrow ^{19}_{13}Al + ^{1}_{0}n}\)
- \(\ce{^{22}_{15}P + ^{1}_{0}n \rightarrow ^{22}_{14}Si + ^{1}_{1}H}\)
Q25.13A
Create the nuclear equation of an isotope of element \(\ce{^{11}O}\) with a mass number of 271 being formed by the bombardment of bismuth-209 by carbon-13. Then form the equation of 6 alpha particle emissions
S25.13A
\(\mathrm{\ce{^{209}_{83}Bi} + 6{^{13}_6C} \rightarrow \ce{^{272}_{89}Ac} + {^1_0n}}\)
\(\mathrm{\ce{^{272}_{89}Ac} \rightarrow 6^4_2He + \ce{^{260}_{77}Ir}}\)
Q25.13B
Write the equation of the creation of the bohrium-255 isotope and 3 beta particles from the bombardment of an iron-48 nucleus with the nucleus of an element with an atomic number of 82 and mass number of 207.
S25.13B
\[\mathrm{\ce{^{207}_{82}Pb} + {^{48}_{22}Fe} \rightarrow {^{255}_{107}Bh} + \ce{3^0_{-1}\beta}}\]
Q25.13C
Write a nuclear equation for the formation of 242-Curium from the bombardment of bismuth-209 with aluminum-27, then followed by an emission of five \(\alpha\)-particles.
S25.13C
\[\ce{^{27}_{13}Al + ^{209}_{83}Bi \rightarrow ^{242}_{96}Cm + 6n}\]
then
\[\ce{^{242}_{96}Cm \rightarrow ^{222}_{86}Rn+5\alpha}\]
To review nuclear reactions, visit the page "Nuclear Chemistry"
Q25.13D
Write out a nuclear equation that show the formation of an isotope element 97 with mass number 255 by the bombardment of bismuth-219 by nickel-64 nuclei followed by a succession of \(\alpha\) particle emission, hence find number of \(\alpha\) particle emission.
S25.13D
\[\ce{^{219}_{83}Bi + ^{64}_{28}Ni \rightarrow ^{255}_{97}Bk + 7\alpha}\]
Q25.21A
The disintegration rate for a sample containing \(\ce{^{90}_{38}Sr}\) as the only radioactive nuclide is 8754 dis h-1. The half life of \(\ce{ ^{90}_{38}Sr}\) is 27.7 years. Estimate the number of \(\ce{^{90}_{38}Sr}\) atoms in the sample at this time.
S25.21A
\(\mathrm{\lambda=\dfrac{0.673}{t_{1/2}}=\dfrac{0.673}{27.7\,y} = 0.0243\,y^{-1}}\)
\(\mathrm{(8754\: dis/h)(8765.81\:h/y)=7.67\times10^7dis\: y^{-1}}\)
\(\mathrm{N=\dfrac{A}{\lambda} = \dfrac{7.67\times10^7\: dis\: y^{-1}}{0.0243\: y^{-1}}= 3.16\times10^9\: atoms}\)
To review half life and activity, visit the page "Radioactive Decay Rates".
Q25.21B
Estimate the number of atoms of potassium-40. When the disintegration rate for the sample containing potassium-40 as the only radioactive nuclide is 700 disintegration per hour and the half life of potassium-40 is 1.28×10^9 years.
S25.21B
\[\mathrm{\lambda = \dfrac{0.693}{1.28\times10^9\,Y}\times\dfrac{1\,Y}{365.25\,d}\times\dfrac{1\,d}{24\,h}=6.1762\times10^{-14}\,h^{-1}}\]
\[\mathrm{N = \dfrac{Rate\:of\:decay}{\lambda}=\dfrac{700\:atoms\times h^{-1}}{6.1762\times10^{-14}\,h^{-1}}=1.133\times10^{16}\,\textrm{Potasium-40}\: atoms}\]
Q25.21C
The rate of disintegration for a sample with \(\ce{^{210}_{85}At}\) is 9871 dis day-1. The half-life of \(\ce{^{210}_{85}At}\) is 4.3 years. Estimate the mass of \(\ce{^{210}_{85}At}\) in the sample.
S25.21C
\(\mathrm{\lambda = \dfrac{0.693}{4.3\,y}\times\dfrac{1\,y}{365.25\,d}=4.41\times10^{-4}\,d^{-1}}\)
\(\mathrm{mass = \dfrac{9871\,atoms/day}{4.41\times10^{-4}\,d^{-1}}\times\dfrac{1\,mole}{6.022\times10^{23}\,atoms}\times\dfrac{210\,grams}{1\,mole}=7.81\times10^{-15}\,g\,present}\)
Q25.21D
The disintegration rate of a sample with \(\ce{^{205}_{83}Bi}\) as the only radioactive nuclide is 3000 dis h-1. The half-life of \(\ce{^{205}_{83}Bi}\) is 15.31 days. Estimate the number of atoms in the sample.
Relevant equations:
\(\mathrm{A=\lambda N}\), \(\mathrm{\lambda = \dfrac{0.693}{t_{1/2}}}\)
S25.21D
Find the decay constant:
\(\mathrm{\lambda = \left(\dfrac{0.693}{15.31\: days}\right) \times \left(\dfrac{1\: day}{24\: hours}\right) = 0.0018860222\: h^{-1}}\)
Rearrange the equation:
\(\mathrm{N=\dfrac{A}{\lambda}}\)
Solve for the number of atoms in the sample:
\(\mathrm{N = \dfrac{3000\: dis\: h^{-1}}{0.0018860222\: h^{-1}} = 1590649.357\: atoms\: \ce{^{205}_{83}Bi}}\) in the sample.
Q25.21E
Estimate the number of \(\ce{^{57}Fe}\) atoms are in the sample given that the half-life of \(\ce{^{57}Fe}\) is 4.8 years and the disintegration rate for a sample containing only \(\ce{^{57}Fe}\) as the only radioactive nuclide is 6650 dis/h
S25.21E
\(\mathrm{\lambda = \left(\dfrac{0.693}{4.8\: years}\right) \left(\dfrac{1\: year}{365.5\: days}\right) \left(\dfrac{1\: day}{24\: hours}\right) = 1.6 \times 10^{-5}/h}\)
\(\mathrm{N = \dfrac{rate\: of\: decay}{\lambda} = \dfrac{6650\: dh^{-1}}{1.6\times10^{-5}\: h^{-1}} = 4.16\times10^8\: Fe\: atoms}\)
Q25.25A
Suppose that a sample containing \(\ce{^{28}_{12}Mg}\) has an activity 1000 times the detectable limit. How long would an experiment have to run with this sample before the radioactivity could no longer be detected?
S25.25A
Given the half-life of \(\ce{^{28}Mg= 21\,h}\),
\[\mathrm{t_{1/2} = \dfrac{0.693}{\lambda}}\]
\[\mathrm{\lambda = \dfrac{0.693}{t_{1/2}}}\]
\[\mathrm{\lambda = \dfrac{0.693}{21\,h} = 0.033\,h^{-1}}\]
or
\[\mathrm{\lambda = \dfrac{0.693}{75600\,s} = 9.16\times10^{-6}\,s^{-1}}\]
We need to find the time it takes to find 1/1000 the initial value. (this being the detectable limit of radiation) using
\[\mathrm{\ln\left(\dfrac{N_t}{N_0}\right) = -\lambda t}\]
\[\mathrm{ln\left(\dfrac{1}{1000}\right) = (0.033\,h^{-1})(t)}\]
\[\mathrm{t = -\dfrac{\ln\left(\dfrac{1}{1000}\right)}{(0.033\,h^{-1})} = 209.33\,h}\]
or in seconds
\[\mathrm{ln\left(\dfrac{1}{1000}\right) = (9.16\times10^{-6}\,s^{-1})(t)}\]
\[\mathrm{t = -\dfrac{\ln\left(\dfrac{1}{1000}\right)}{(9.16\times10^{-6}\,s^{-1})} = 754121\,s}\]
To review half life and activity, visit the page "Radioactive Decay Rates".
Q25.25B
If a sample containing \(\ce{^{33}_{16}S}\) is having activity 100 times the detectable limit. How long will the experiment need to be run for this sample before the radioactivity could not detected? Assume half life of \(\ce{^{33}_{16}S}\) is 14.3 day.
S25.25B
\(\ce{^{33}_{16}S}\) half life is given. Since we need to determine the time require getting to the detectable limit. \(\dfrac{1}{100}\) of the initial value. Hence we use
\[\mathrm{\lambda = \dfrac{0.693}{t_{1/2}}=\dfrac{0.693}{14.3\, d}=0.0485\,d^{-1}}\]
\[\mathrm{\ln \left (\dfrac{1}{100}\right) =-0.0485\,d^{-1}\left ( t \right )}\]
\[\mathrm{T=\dfrac{\ln\left(\dfrac{1}{100}\right )} {-0.0485\,d^{-1}}=94.95=95\,days}\]
Q25.25C
Suppose a sample with \(\ce{^{170}_{69}Tm}\) has an activity 100 times the detectable limit. How long until the sample's radioactivity is no longer detectable?
S25.25C
\(\mathrm{\ce{^{170}_{69}Tm}\: half\: life = 128.6\: days}\)
\(\mathrm{\lambda = \dfrac{0.693}{t_{1/2}}=\dfrac{0.693}{128.6\,d}=0.00539\,d^{-1}}\)
\(\mathrm{\ln\dfrac{1}{100} = -0.00539\,d^{-1}(t)}\)
\(\mathrm{t=854\: days}\)
Q25.25D
Suppose the activity of a sample containing \(\ce{^{35}S}\) has an activity 500 times the detectable limit. How long before the radioactivity can no longer be detected if the experiment is run continuously?
S25.25D
\(\mathrm{^{35}_{16}S\:\: t_{1/2} = 88\,d}\)
\(\mathrm{\lambda = \dfrac{0.693}{t_{1/2}} = \dfrac{0.693}{88\,d} = 0.007875}\)
\(\mathrm{\ln\left(\dfrac{1}{500}\right) = -0.007875\,d^{-1}(t)}\)
\(\mathrm{t=789.16\: days}\)
Q25.25E
Suppose that a sample of \(\ce{^{23}Mg}\) (\(\mathrm{\textrm{Half-life} = 11.32\, s}\)) has an activity 500 times the detectable limit. How long could experiments be run before radiation falls below detectable limits?
S25.25E
In this problem, we are trying to determine the time necessary to reach undetectable amounts of radiation, which is \(\mathrm{\left(\dfrac{1}{500}\right)}\) the initial value.
Determine the rate constant:
\(\mathrm{\lambda = \dfrac{0.693}{11.32\, s} = 0.0612}\)
Use the equation relating rate of decay to amount of sample and time:
\(\mathrm{\ln(1) - \ln(500) = -(0.0612190813\, s^{-1})(t)}\)
Resulting in:
\(\mathrm{t = 101.5142332\, s}\)
Q25.27A
As the museum authenticator, you have been given the task of determining which era a recently donated piece of pottery is from. If, through radiocarbon dating, you find that the disintegration rate is 13 dis min-1 g-1, what is the age of the pottery piece?
S25.27A
Original rate of disintegration: 15 dis min-1 g-1 & \(\mathrm{\textrm{half-life} = 5730\, y}\)
\(\mathrm{\lambda = \dfrac{0.693}{5730\,y}=1.21\times10^{-4}\,y^{-1}}\)
\(\mathrm{\ln\dfrac{13\, dis/min}{15\, dis/min}=-\lambda t = -1.21\times10^{-4}\, y^{-1} (t)}\)
\(\mathrm{t=1182\, years}\)
Q25.27B
A museum has just bought an ancient Greek vase from a collector. Radiocarbon dating of the vase reveals a distintegration rate of 8.0 dis min-1 g-1. The original disintegration rate is 15.0 dis min-1 g-1. If the vase was from the "Golden Age" of Athens around 480 BC-404 BC do you think the object is authentic? Explain.
S25.27B
\(\mathrm{\ln\dfrac{N_t}{N_0} = -\lambda t}\)
\(\mathrm{t_{1/2} = \dfrac{\ln 2}{\lambda}}\)
\(\mathrm{\lambda = \dfrac{\ln 2}{t_{1/2}}= \dfrac{\ln 2}{5730\:years} = 1.21 \times 10^{-4}\: years^{-1}}\)
\(\mathrm{\ln\dfrac{8.0\: dis\: min^{-1}\: g^{-1}}{15\: dis\: min^{-1}\: g^{-1}} = -(1.21 \times 10^{-4}\: years^{-1})t}\)
\(\mathrm{t = \dfrac{\ln\left(\dfrac{8.0\: dis\: min^{-1}\: g^{-1}}{15\: dis\: min^{-1}\: g^{-1}}\right)}{-(1.21 \times 10^{-4}\: years^{-1})} = 5195.11\: years}\)
Since the "Golden Age" of Athens was in 480 BC-404 BC, the vase could be authentic.
To review radioactive decay rates, please visit the page "Radioactive Decay Rates".
Q25.27C
A piece of wood is claimed to found in Qin Mausoleum and is offered for sale to an art museum. Radiocarbon dating of the object reveals a disintegration rate of 5.0 dis min-1 g-1. Do you think the object is real? Explain your answer. Given that the \(\mathrm{\lambda = \dfrac{0.693}{t_{1/2}}=\dfrac{0.693}{5730\,y}=1.21\times10^{-4}\,y^{-1}}\) and the initial rate of decay is about 15 dis/min.
S25.27C
Use \(\mathrm{\lambda = \dfrac{0.693}{t_{1/2}}=\dfrac{0.693}{5730\,y}=1.21\times10^{-4}\,y^{-1}}\)
\(\mathrm{\ln\dfrac{5\, dis/min}{15\, dis/min} = -1.0986\,s=-\lambda t}\)
\(\mathrm{t = \dfrac{1.0976}{1.21\times10^{-4}\,y^{-1}}=9071\,years}\)
The object is around 9000 years old, and it is probably not from the Qin Mausoleum, which is around 3000B.C.
Q25.27D
- Calculate the energy of the alpha decay of \(\ce{^{212}Po}\) given the following atomic mass and conversion factors:
\(\mathrm{\underset{\Large{209.0231\,u}}{^{212}_{84}Po} \rightarrow \underset{\Large{207.2211\,u}}{^{208}_{82}Pb} + \underset{\Large{4.0026\,u}}{^4_2He}}\)
\(\mathrm{1\,u=1.4924 \times 10^{-10}J}\) and \(\mathrm{1\,u= 931.5\, MeV}\)
- How much energy, in megaelectronvolts, would be released if 3 alpha particles were destroyed?
S25.27D
- \(\mathrm{\Delta m = (mass\: of\: ^{208}Pb + ^4He) - (mass\: of\: ^{212}Po)}\)
\(\mathrm{\Delta m= 207.2211\,u + 4.0026\,u - 209.0231\,u = 2.2006\, u}\)
\(\mathrm{E= 2.2006\, u\, (1.4924 \times 10^{-10}\,J/u)}\)
\(\mathrm{E= 3.284 \times 10^{-10}\, J}\)
- \(\mathrm{^4_2He = alpha\: particle}\)
\(\mathrm{= 4.0026\, u}\)
\(\mathrm{= 3 \times 4.0026\, u = 12.0078\, u}\)
\(\mathrm{12.0078\, u \times \left(\dfrac{931.50\, MeV}{1\, u}\right) = 11185\, MeV}\)
*note that conversation factors are derived from equation \(\mathrm{E=mc^2}\).
Q25.27E
Approximately how old is a wooden object given that the object has a disintegration rate of 11.0 dis/minxg? It is given that the half-life of this particular wood is 5730 years.
S25.27E
\(\mathrm{\lambda = \dfrac{0.693}{5730\:years} = 1.21\times10^{-4}}\)
\(\mathrm{\ln\left(\dfrac{11\:dis/min}{15\:dis/min}\right) = -0.310155 = -\lambda t}\)
\(\mathrm{t = \dfrac{0.310155}{1.21\times10^{-4}\,y^{-1}}=2565.26\: years}\)
Q25.27F
A wooden atlatl is discovered sitting atop a glacier in the Netherlands as the ice recedes. Since relative stratification is not an option in dating the ancient device, archaeologists use radiocarbon dating to reveal a decay rate of 15 dis h-1. A recent sample of freshly cut wood of the same type reveals a decay rate of 30 dis h-1. What is the age of the atlatl?
S25.27F
The problem asks us to determine the age of a recently exposed atlatl (a simple throwing device used to hurl spears or rocks with greater torque than that of the human arm) by using radiocarbon dating.
Knowing that the \(\mathrm{\textrm{half-life of Carbon-14} = 5730\, years}\), we can use these equations:
\[\mathrm{\lambda = \dfrac{0.693}{t_{1/2}}}\]
\[\mathrm{\ln\left(\dfrac{N_t}{N_0}\right) = - \lambda t}\]
Find the rate constant:
\[\mathrm{\lambda = \dfrac{0.0693}{57304} = 1.21\times10^{-4}}\]
Date the material:
Knowing that a recently cut material has a decay rate of 30 dis h-1, we can compare to the found atlatl.
\[\mathrm{\ln(15) - \ln(30) = -(1.21\times 10^{-4}\,y^{-1})(t)}\]
\[\mathrm{t = 5728.48\, y \sim 5700\, y}\]
The atlatl is approximately 5700 years old.
Q25.29A
What should be the mass ratio of \(\ce{^{210}Po/^{238}U}\) in an object that is around 2.4X109 years old? The half-life of \(\ce{^{238}U}\) is 4.47X109 years. [Hint: one \(\ce{^{210}Po}\) atom is final decay product of one \(\ce{^{238}U}\) atom]
S25.29A
Determine the decay constant using the equation \(\mathrm{\lambda = \dfrac{A}{N}}\)
\[\mathrm{\dfrac{0.693}{4.47\times10^9\, y} = 1.55\times10^{-10}\,y^{-1}}\]
Determine the ratio of (\(\mathrm{N_p}\)), number of \(\ce{Pa}\) atoms after 2.4X109 y, to \(\mathrm{N_o}\), initial number of \(\ce{Pa}\) atoms \(\mathrm{\ln\dfrac{N_p}{N_o} = -kt}\)
\[\mathrm{-(1.55\times10^{-10}\,y^{-1})(2.4\times10^9\,y) = -0.372}\]
\[\mathrm{e^{-0.372}=0.689}\]
\[\mathrm{\dfrac{N_p}{N_o} = 0.689}\]
For every mole of \(\ce{^{238}U}\) present initially, after 2.9X109y, there are 0.689 moles of \(\ce{^{238}U}\) and 0.310 moles of \(\ce{^{210}Po}\)
Computing mass ratio
\[\mathrm{\dfrac{0.310\, mol\, ^{210}Po}{0.689\, mol\, ^{238}U}\times\dfrac{1\, mol\, ^{238}U}{238\,g\, U}\times\dfrac{210\,g\, Po}{1\, mol\, ^{210}Po}}\]
\[\mathrm{= \dfrac{65.1\,g\: ^{210}Po}{166.12\: ^{238}U}}\]
\[\mathrm{= \dfrac{0.392\,g\: ^{210}Po}{1\,g\: ^{238}U}}\]
For review on this topic, visit the page "Radioactive Decay Rates" and "Decay Pathways"
Q25.29B
Calculate the binding energy per nucleon, in megaelectronvolts, of the nuclide \(\ce{^{30}_{14}Si}\) if its measured mass is 28.1900 u.
\(\mathrm{Mass\: of\: proton=1.0073\, u}\)
\(\mathrm{Mass\: of\: neutron= 1.0087\, u}\)
S25.29B
Video Solution
\(\mathrm{(14\: protons \times 1.0073\, u) + (16\: neutrons \times 1.0087\, u) + (14\: electrons \times 0.00054858\, u)= 30.249\, u}\)
Mass Defect:
\(\mathrm{30.249\, u - 28.1900\, u = 2.059\, u}\)
\(\mathrm{2.059\, u \times \left(\dfrac{931.5\, MeV}{1\, u}\right)= 1918\, MeV/nucleus}\)
\(\mathrm{\dfrac{1918\, MeV/nucleus}{\textrm{30 (total neutrons and protons)}}= \textrm{63.932 MeV per nucleon}}\)
Q25.29C
A science fiction writer is writing a short story about a team of scientists landing on Jupiter's moon for the first time. In their exploration, they find a large obelisk with some kind of electromagnetic field powered by an ancient fission reactor. Since the scientists' space suits already protect against the harmful radiation of interplanetary space, they decide to collect a sample and use it to find an approximate absolute age for the ship. The scientists determine that the reactor was able to run efficiently using proactinium-231 as fuel (remember that this is, indeed, fiction). If the scientists report to mission control that the reactor has been running for 30,000 years, what ratio of proactinium-231 to Uranium-235 and its derivatives was observed in the fictional field laboratory?
S25.29C
The half life of Proactinium-231 is 32,760 years. We use \(\mathrm{\lambda = \dfrac{0.693}{t_{1/2}}}\) to determine the rate constant of the reaction.
\[\mathrm{\lambda = \dfrac{0.693}{32,760\, y} = 2.11\times10^{-5}\, y^{-1}}\]
Now, using the equation: \(\mathrm{\ln\left(\dfrac{N_t}{N_0}\right) = - \lambda t}\), we can determine the ratio of moles of the protactinium-231 to Uranium-235 (and its derivatives)
\(\mathrm{\ln\left(\dfrac{N_t}{N_0}\right) = - \lambda t \Rightarrow \left(\dfrac{N_t}{N_0}\right) = e ^{- \lambda t} \Rightarrow \left(\dfrac{N_t}{N_0}\right) = e^{-(2.11\times10\textrm{E-005})(30,000)} = 0.53}\)
Knowing that the molar ratio of Proactinium-231 to Uranium-235 (and derivatives) is 0.53, we can determine the mass ratio of the sample collected fictional scientists.
Since there is a ratio of 0.53, that means there is 0.53 proactinium-231 moles per 1 mole of sample and conversely that there are 0.47 moles of Uranium-235 and its derivatives for every 1.00 mole of sample.
So, using some conversion factors for the molar mass of the species, we can determine that the ratio of Proactinium-231 to Uranium-235 was:
\(\mathrm{\left[\dfrac{\textrm{0.47 moles U-235}}{\textrm{0.53 moles Pa-231}}\right] \times \left[\dfrac{\textrm{235 g of U-235}}{\textrm{1 mole of U-235}}\right] \times \left[\dfrac{\textrm{1 mole of Pa-231}}{\textrm{231 g Pa-231}}\right] = \dfrac{\textrm{110.45 g U-235}}{\textrm{122.43 g Pa-231}} = \dfrac{\textrm{1 g U-235}}{\textrm{1.108 g Pa-231}}}\).
If the fictional Scientists reported that the artifact they found was 30,000 years old, they would have found 1 g of U-235 and its derivatives for every 1.108 g of Pa-231 fuel in the reactor.
Q25.29D
\(\mathrm{^{209}Bi/ ^{231}Pa}\) is in a meteorite approximately 2.6 x 109 years old. The half-life of \(\ce{^{231}Pa}\) is 1.21 x 10 10 years. What should the mass ratio be of \(\mathrm{^{209}Bi/ ^{231}Pa}\)?
S25.29D
\[\mathrm{\lambda = \dfrac{0.693}{t_{1/2}}}\), \(\mathrm{t_{1/2}= 1.21\times10^{10}\: years}\]
\[\mathrm{\lambda = \dfrac{0.693}{1.21\times10^{10}\,years} = 5.73 \times 10^{-11}\,years}\]
\[mathrm{\ln\left(\dfrac{N_t}{N_o}\right) = -kt = (-5.73\times10^{-11})(2.6\times10^9) = -0.14898}\]
\[\mathrm{\dfrac{N_t}{N_o}=0.861586}\]
Thus for every mol of \(\ce{^{231}Pa}\) present initially, there is 0.861 mol \(\ce{^{231}Pa}\) and 0.139 mol \(\ce{^{209}Bi}\).
\[\mathrm{\left(\dfrac{0.159\, mol\, ^{209}Bi}{0.861\, mol\, ^{231}Pa}\right)\left(\dfrac{209\,g}{1\,mol\, ^{209}Bi}\right)\left(\dfrac{1\, mol\, ^{231}Pa}{231\,g}\right) = \left(\dfrac{0.167\,g\, ^{209}Bi}{1\,g\, ^{231}Pa}\right)}\]
Q25.29E
An asteroid is about 7.1x107 years old and contains \(\ce{^{208}Pb}\) and \(\ce{^{232}Th}\). What should the mass ratio \(\ce{^{208}Pb/^{232}Th}\) be? (The half-life of \(\ce{^{232}Th}\) is 1.39x1010 years)
S25.29E
The constant k \(\mathrm{\ = 4.99\times 10^{-11}\, y^{-1}}\)
Then plug in the equation ln N1/N0 = -kt
\(\mathrm{\ln\dfrac{N_t}{N_0} = -kt = -(4.99\times 10^{-11}\, y^{-1})(7.1\times10^7\,y)=-0.0035}\)
\(\mathrm{\dfrac{N_t}{N_0} = 0.996}\)
Also, we know that the atom mass of Th is 232 and the atom mass of Pb is 208.
\(\mathrm{\dfrac{0.004\: mol\, ^{208}Pb}{0.996\: mol\, ^{232}Th} \times \dfrac{1\, mol\,^{232}Th}{232\,g\,^{232}Th} \times \dfrac{208\,g\,^{208}Pb}{1\,mol\,^{208}Pb} = \dfrac{0.0036\,g\,^{208}Pb}{1\,g\,^{232}Th}}\)
Therefor, the mass of 208Pb/232Th is 0.0036g 208Pb/ 1g 232 Th.
Q25.29F
What should be the mass ratio of \(\ce{^{203}_{81}Tl/^{236}_{93}Np}\) in a meteorite that is about 3×109 years. Assume the half life of \(\ce{^{203}_{81}Tl}\) is 2×1010 years and one \(\ce{^{203}_{81}Tl}\) atom is the final decay product of one \(\ce{^{236}_{93}Np}\) atom.
S25.29F
First we determine the decay constant. \(\mathrm{\Lambda = \dfrac{0.693}{t_{1/2}}= \dfrac{0.693}{2\times10^{10}}=3.463\times10^{-11}\,y^{-1}}\)
Then we can determine the ratio of the (Nt), the number of the Tl atoms after 3 × 109 years, to (N0), the initial number of Tl atoms:
\(\mathrm{\ln\left (\dfrac{N_t}{N_0} \right )=-kt - (3.463\times10^{-11}\,y^{-1})(3\times10^9)=-0.10389}\)
\(\mathrm{\dfrac{N_t}{N_0}=0.901}\)
Thus for every mole of \(\ce{^{203}_{81}Tl}\) present initially, after 3 × 109 there are 0.901 mole and 0.09 mole \(\ce{^{203}_{81}Tl}\). From this information, we can compute the mass ratio:
\(\mathrm{\dfrac{0.12\:mol\:^{203}_{81}Tl}{0.901\:mol\:^{236}_{93}Np} \times\dfrac{203\:g\:^{203}_{81}Tl}{1\:mol\:^{203}_{81}Tl} \times\dfrac{1\:mol\:^{236}_{93}Np}{236\:g\:^{236}_{93}Np} =\dfrac{24.36\:g\:^{203}_{81}Tl}{212.636\:g\:^{236}_{93}Np}}\)
Q25.33A
Using Einstein's famous equation \(\mathrm{E=mc^2}\) determine the amount of energy released from the destruction of 6.542x10-19g.
S25.33A
First convert g to kg to get the correct units for joules. Since the \(\mathrm{units\: of\: joules= \dfrac{kg\,m^2}{s^2}}\)
\[\mathrm{6.542\times10^{-19}\,g \times \dfrac{1\,kg}{1000\,g} = 6.542\times10^{-22}\,kg}\]
\[\mathrm{c=2.9998\times10^8\textrm{ m/s speed of light}}\]
\[\mathrm{E=(6.542\times10^{-22}\,kg)(c=2.9998\times10^8\: m/s)^2= 5.887\times10^{-5}\textrm{ Joules of energy}}\]
To review Einstein's equation, please visit the page "Fission and Fusion "
Q25.33B
Use proper equations in the text to determine the energy in kilojoules correspond to destruction of 6 × 10-23 grams of matter
S25.33B
\[\mathrm{E=6\times10^{-23}\,g\times\left (\dfrac{1\,Kg}{1000\,g} \right )\times \left(3.00\times10^8\,\dfrac{m}{s}\right)^2=5.42\times10^{-9}\,kg\,m^2\,s^{-2}}\]
\[\mathrm{=5.42\times10^{-9}\,J}\]
We are asked to calculate the energy as 6*10^23 grams of matter are destroyed. Clearly this is a nuclear reaction because matter is undergoing a change in composition where atoms are rearranged due to this destruction of matter that the problem mentions. In nuclear reactions, we know that matter undergoes a transformation in which new matter is made. This means that atoms are not conserved which also means that mass is not conserved.
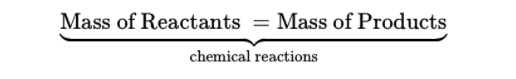
We can then use Einstein's mass-energy equivalence, E=mc^2, which relates energy and mass in nuclear equations.
The above equation is done correctly. We must substitute 6*10^23 grams of matter in for m, mass. However, we must convert grams to kilograms since we are multiplying by constant c^2 which has units labeled as m^2s^-2. This is because energy is labeled as Joules, J. Joules are equal to kg*m^2*s^-2, NOT grams.
As we multiply and divide accordingly, we find that E= 5.42*10^-9 Joules of energy. However, we are not finished with the problem yet. We are asked to find the amount of energy in KILOjoules. Not Joules. To find this, we simply multiply 5.42*10^-9 Joules by 1000kj since 1J=1000kj. Our final result is 5.42*10^-6 kj of energy.
Q25.33C
What is the energy (in joules) associated with the destruction of 9.56x10-24 g of matter?
S25.33C
\(\mathrm{E=mc^2}\)
\(\mathrm{E = 9.56\times10^{-24}\,g \times\dfrac{1\,kg}{10^3\,g}\times(3.00\times10^8\, m/s)^2 = 2.87\times10^{-18}\,kg\, m^2\, s^2 = 2.87 \times 10^{-18}\,J}\)
Q25.33D
How much energy is released, in megaelectronvolts, in the nuclear reaction:
\[\ce{^{27}_{13}Al + ^4_2He \rightarrow ^{30}_{15}P + ^1_0n}\]
- \(\mathrm{Al\: isotope= 27.021\, u}\)
- \(\mathrm{Alpha\: particle= 4.0026\, u}\)
- \(\mathrm{Phosphorus= 30.9721\, u}\)
- \(\mathrm{Neutron = 1.0087\, u}\)
S25.33D
\[\mathrm{\Delta m= (^{27}Al + alpha) - (^{30}Phosphorus + Neutron)}\]
\[\mathrm{\Delta m= (27.021\,u + 4.0026\,u) - (30.9721\,u + 1.0087\,u)}\]
\[\mathrm{\Delta m= -0.9572\, u}\]
\[\mathrm{-0.9572\, u \times \left(\dfrac{931.5\, MeV}{1\, u}\right)= -891.63\, MeV}\]
Q25.33E
Determine the amount of energy involved in the destruction of 5.98 x 10-22 g of matter
S25.33E
\[\mathrm{E - \left(5.98\times10^{-22}\,g\right)\left(\dfrac{1\,kg}{1000\,g}\right) \times (3.00\times10^5\,m/s)^2 = 5.38 \times 10^{-8}\,kg\,m^2/s^2 = 5.38 \times 10^{-8}\, J}\]
Q25.33F
Determine the energy of the destruction of 1.00g of matter.
S25.33F
The vagueness of the problem should be a strong indication that we have a relatively general set of skills with which to find a solution. In this case, we use the mass energy equivalence to find the Energy released from the full conversion of 1.00g of matter.
\(\mathrm{E=mc^2}\)
\(\mathrm{E = 1.0\, g \times \left(\dfrac{1\,kg}{1000\, g}\right) \times (3.00\times10^8)^2 = 9.00 \times 10^{13}\, kg\, m^2\, s^{-2} = 9.00 \times 10^{13}\, J}\)
Q25.37A
Calculate the energy released in the reaction (in megaelectronvolts)
\[\ce{^{15}_7N + ^4_2He \rightarrow ^{18}_8O + ^1_1H}\]
The masses are
- \(\ce{^5_7N} = \mathrm{15.00011\: u}\)
- \(\ce{^4_2He} = \mathrm{4.00260\: u}\)
- \(\ce{^{18}_8O} = \mathrm{17.99916\: u}\)
- \(\ce{^1_1H} = \mathrm{1.00783\: u}\).
S25.37A
\(\mathrm{[1.00783\: u-mass\: e^-]+[17.99916\: u-(8\times mass\: e^-)]-[4.00260\: u-(2\times mass\: e^-)]-[15.00011\: u-(7\times mass\: e^-)] =}\)
\(\mathrm{1.00783\: u + 17.99916\: u - 4.00260\: u - 15.00011\: u - 9\: e^- + 9\: e^- = 0.00428\: u}\)
\(\mathrm{0.00428\: u \times (931.5\: MeV/u) = 3.99\: MeV}\)
For review on this topic, visit the page "Decay Pathways" .
Q25.37B
Calculate the energy in MeV, released in the below nuclear reaction
\[\ce{^{6}_{3}Li + 2(^{4}_{2}He) \rightarrow ^{13}_{6}C + ^1_1H}\]
The nucleic mass
- \(\mathrm{^{6}_{3}Li=6.01514\: u}\),
- \(\mathrm{^{13}_{6}C=13.00335\: u}\),
- \(\mathrm{^{1}_{1}H=1.00783\: u}\), and
- \(\mathrm{^{4}_{2}He=4.0026\: u}\).
S25.37B
Video Solution
\[\mathrm{Mass\:defect=(6.01514+(2\times4.0026))-(13.0035+1.00783)=0.00901\:u}\]
\[\mathrm{Energy=0.00901\:u\times\dfrac{931.5\:MeV}{1\:u}=4.06\:MeV}\]
Q25.37C
How much energy, in megaelectronvolts, is released in the following nuclear reaction?
\(\mathrm{\ce{^{241}_{95}Am} \rightarrow \ce{^{237}_{93}Np} + {^4_2He}}\)
The nuclidic masses are \(\mathrm{\ce{^{241}_{95}Am}=241.056829\, u}\), \(\mathrm{\ce{^{237}_{93}Np}=237.0481734\, u}\), and \(\mathrm{^4_2He=4.00260\, u}\).
S25.37C
\(\mathrm{mass\: defect=(241.056829\,u)-(237.0481734\, u + 4.00260\,u) = 0.0060556\, u}\)
\(\mathrm{Energy=0.0060556\, u \times \dfrac{931.5\, MeV}{1\,u} = 5.641\,MeV}\)
Q25.37D
Predict if each of the following radionuclides decays by beta or positron emission:
- \(\ce{^{29}Si}\)
- \(\ce{^{27}Si}\)
- \(\ce{^{112}Sn}\)
- \(\ce{^{120}Sn}\)
S25.37D
Recall that Beta emissions result in a gain of protons via an effective \(\ce{_{-1}\beta^-}\) particle, while a positron emission lead to a decrease in protons due to an effective \(\ce{_{+1}\beta^+}\) charge. This is ignoring emitted neutrinos of course
To become stable, the nucleus needs to get on the belt of stability:
For lower atomic numbers, the number of neutrons and protons should be about equal.
- \(\ce{^{29}Si}\) Beta emission to gain protons and equal the neutrons available
- \(\ce{^{27}Si}\) Positron emission to lose protons and equal the neutrons available
For larger atomic numbers, however, a nucleus would require a higher number of neutrons in order to keep the atom stabilized, therefore increasing the Neutron/Proton ratio.
- \(\ce{^{120}Sn}\) Beta emission to gain protons to gain stability
- \(\ce{^{112}Sn}\) Positron emission to lose protons to gain stability
Q25.37E
Calculate the energy (in Megaelectronvolts) released in the nuclear reaction:
\[\mathrm{\ce{^{14}_7N} + {^4_2He^{2+}} \rightarrow \ce{^{17}_8O} + {^1_1H}}\]
S25.37E
Mass defect:
\(\mathrm{(\textrm{Nitrogen-14} + alpha\: particle) - (\textrm{Oxygen-17} + Proton) = (4.00260 + 14.00307) - (1.00783 + 16.99913) = -0.00129\,u}\).
The total change in mass is 0.00129u.
\(\mathrm{Energy\: released = 931 \times (0.00129\,u) = 1.20\, MeV}\)
Q25.37F
Calculate energy released in nuclear reaction: \(\mathrm{\ce{^{11}_6C} + {^4_2He} \rightarrow \ce{^{14}_7N} + {^1_1H}}\)
mass of nuclei:
\(\mathrm{\ce{^{11}_6C} = 10.9\,\mu}\)
\(\mathrm{^4_2He = 3.21\,\mu}\)
\(\mathrm{\ce{^{14}_7N} = 13.104\,\mu}\)
\(\mathrm{^1_1H = 1.01\,\mu}\)
S25.37F
\(\mathrm{(13.104\,\mu + 1.01\,\mu) - (10.9\,\mu + 3.21\,\mu) = 0.004\,\mu}\)
\(\mathrm{0.004\,\mu\left(\dfrac{931.5\,MeV}{1\,\mu}\right) = 3.71\, MeV}\)
Q25.41A
Which member of the following pairs of nuclides would you expect to be the most abundant in natural sources:
- \(\ce{^{12}_6C}\) or \(\ce{^{14}_6C}\)
- \(\ce{^{18}_8O}\) or \(\ce{^{19}_8O}\)
Explain your reasoning.
S25.41A
- \(\ce{^{12}_6C}\), the neutron to proton ratio is 1-to-1, (6 protons and 6 neutrons) which alludes to stability for elements with atomic number less than 20.
- \(\ce{^{18}_8O}\), even though there is no 1-to-1, this isotope has an even number of both protons and neutrons (8 protons to 10 neutrons). Which is associated with being a stable isotope.
For review on this topic, visit the page "Decay Pathways" .
Q25.41B
Which member of the following pairs of nuclide would you expect most abundant in natural sources. Explain your answer
- Ar-40 or Ar 42
- Cl-36 or Cl-38
S25.41B
- Ar-40 is more abundant , because it is closer to the average atomic mass of Ar, which is 39.95.
- Cl-36 is more abundant, because it is closer to the average atomic mass of Cl, which is 35.45.
Q25.41C
Which isotope is more likely to be the most abundant in nature? Explain.
- \(\ce{^{36}_{18}N}\) vs. \(\ce{^{38}_{18}N}\)
- \(\ce{^{89}_{38}Sr}\) vs. \(\ce{^{90}_{38}Sr}\)
S25.41C
- \(\ce{^{36}_{18}N}\) would be more abundant (more stable) because a 1-to-1 ratio of protons-to-neutrons is most favorable in elements with an atomic number less than 20.
- \(\ce{^{90}_{38}Sr}\) is probably more abundant (more stable) because isotopes with an even number of protons and an even number of neutrons are typically favored.
Q25.41D
Which nuclide is more common:
- \(\ce{^9Be}\) or \(\ce{^6Be}\)?
- \(\ce{^{35}Cl}\) or \(\ce{^{43}Cl}\)
S25.41D
- \(\ce{^9Be}\) is the more common isotope. The other isotope of Beryllium given is radioactive, which is a good indication that it is the less stable species. Additionally, \(\ce{^9Be}\) has more neutrons than protons whereas \(\ce{^6Be}\) has the same number.
- \(\ce{^{35}Cl}\) is, indeed, the most common isotope of Chlorine that occurs naturally. It nearly has a proton to neutron ration of 1-to-1 which is generally favored in elements with atomic numbers less than or equal to 20. More so, \(\ce{^{43}Cl}\) is highly radioactive and decays almost immediately.
Q25.41E
Calculate the following questions regarding \(\ce{^{35}S}\) isotope, given its half life is 88 days:
- What is the decay constant for \(\ce{^{35}S}\) (in s-1)?
- How many atoms will disintegrate per second (the activity) of a 2.00 mg sample of \(\ce{^{35}S}\) sample?
- After 176 days, what mass of the \(\ce{^{35}S}\) of the initial 2.00 mg sample will remain?
- What is the sample's rate of radioactive decay after 176 days?
S25.41E
Video Solution
- Recall that: \(\mathrm{\lambda= \dfrac{\ln 2}{t_{1/2}}}\)
Therefore, we need to calculate the decay rate, and convert it into s-1
\(\mathrm{\lambda= \left(\dfrac{0.693}{88\,d}\right) \times \left(\dfrac{1\, d}{24\, h}\right) \times \left(\dfrac{1\, h}{60\, mins}\right) \times \left(\dfrac{1\, min}{60\, s}\right)= 9.11 \times 10^{-8}\, s^{-1}}\)
- We need to convert the 2.00 mg sample into atoms:
\(\mathrm{0.00200\,g \times \left(\dfrac{1\, mol\: ^{35}S}{32.07\, g}\right) \times \left(\dfrac{6.022 \times 10^{23}\: ^{32}P\, atoms}{1\: mol\: ^{32}P}\right)= 3.75 \times 10^{19}\, atoms}\)
Since: \(\mathrm{activity = \lambda N}\)
\(\mathrm{\lambda N= 9.11 \times 10^{-8}\,s^{-1} \times 3.75 \times 10^{19}\: atoms}\)
\(\mathrm{\lambda N= 3.42 \times 10^{12}\, atoms/s}\)
- To see how much of mass is left after a certain time period, recall that a half life is a time that indicates how much a material will decrease by one half of its initial quantity. Therefore, we need to calculate how many half lives the sample undergoes:
\(\mathrm{\dfrac{176}{88\,d}= 2\: half\: lives}\)
Since the sample decreases by 1/2 for each half life, we put one half to the power of each half life:
\(\mathrm{mg\: ^{35}S = 2.00\: mg \times \left(\dfrac{1}{2}\right)^2= 2.00 \times \dfrac{1}{4}= 0.5\: mg\: ^{35}S}\)
- Rate of decay is proportional to \(\ce{N}\), while: \(\mathrm{activity = \lambda N}\)
\(\mathrm{Rate\: of\: decay= \dfrac{1}{4} \times activity}\)
\(\mathrm{Rate\: of\: decay= \dfrac{1}{4} \times 3.42 \times 10^{12}\, atoms/s = 8.55 \times 10^{11}\, atoms/s}\)
Q25.41F
Of the following pairs of nuclides, which do you expect to be more abundant in natural resources? Why?
- \(\ce{^{24}_{12}Mg}\) or \(\ce{^{26}_{12}Mg}\)
- \(\ce{^{21}_{10}Ne}\) or \(\ce{^{22}_{10}Ne}\)
- \(\ce{^{10}_5B}\) or \(\ce{^{11}_5B}\)
S25.41F
- \(\ce{^{24}_{12}Mg}\) because a 1 to 1 proton neutron ratio is associated with stability for atoms with \(\mathrm{z<20}\)
- \(\ce{^{22}_{10}Ne}\) because an even number of neutrons is associated with stability
- \(\ce{^{11}_5B}\) because although the ratio for \(\ce{^{10}_5B}\) is closer to I, the even number of neutrons is more associated with stability
Q25.42A
Which nuclide of the pair is more abundant?
- \(\ce{^{35}Ar}\) or \(\ce{^{40}Ar}\)
- \(\ce{^{132}Xe}\) or \(\ce{^{122}Xe}\)
- \(\ce{^{56}Fe}\) or \(\ce{^{61}Fe}\)
- \(\ce{^{51}V}\) or \(\ce{^{53}V}\)
S25.42A
- \(\ce{^{40}Ar}\) is more abundant, since it is not radioactive like \(\ce{^{35}Ar}\). It also has a proton to neutron ratio of 1-to-1, which is usually favored in species with atomic number less than or equal to 20.
- \(\ce{^{132}Xe}\) is more stable, which is determinable from its lack of radioactivity and its proximity in mass to the periodic table average.
- \(\ce{^{56}Fe}\) is the most abundant, which is discernible from the fact that it is not radioactive (unlike \(\ce{^{61}Fe}\)). More prominently, \(\ce{^{61}Fe}\) has an odd number of neutrons but an even number of protons, making it inherently unstable for its size.
- \(\ce{^{51}V}\) is more abundant. \(\ce{^{53}V}\) is radioactive and thus unstable.
Q25.42B
Which isotope is more likely to be the most abundant in nature? Explain.
- \(\ce{^{14}_{6}C}\) vs. \(\ce{^{12}_{6}C}\)
- \(\ce{^{35}_{17}Cl}\) vs. \(\ce{^{36}_{17}Cl}\)
- \(\ce{^{96}_{44}Ru}\) vs. \(\ce{^{95}_{44}Ru}\)
S25.42B
- \(\ce{^{12}C}\) would be more abundant because it is more stable. It is more stable because of its 1-to-1 ratio of neutrons-to-protons that is more favorable in elements with \(\mathrm{Z\leq 20}\)
- Solution: \(\ce{^{35}Cl}\) should be more abundant. Both have an odd number of protons, but \(\ce{^{35}Cl}\) has an even number of neutrons, which is more stable in nature.
- Solution: \(\ce{^{96}Ru}\) should be more abundant. An even number of neutrons and an even number of protons are usually more stable in nature.
Q25.42C
Which members of the following pairs of nuclides would you expect to be most abundant in natural sources: a) \(\ce{^{32}_{16}S}\) or \(\ce{^{34}_{16}S}\) b) \(\ce{^{70}_{33}As}\) or \(\ce{^{73}_{33}As}\) c) \(\ce{^{182}_{74}W}\) or \(\ce{^{189}_{74}W}\) Explain why.
S25.42C
- \(\ce{^{32}_{16}S}\) is more stable than \(\ce{^{34}_{16}S}\). Since the number of protons and number of neutrons in both are even, this will not determine which is more stable. Since there are no magic numbers of protons or neutrons, this will not determine which is more stable. Thus, you must calculate the ratio of neutrons to protons, which in this case is 1:1 for \(\ce{^{32}_{16}S}\). This ratio is consistent with the fact that for \(\mathrm{Z<20}\), the ratio closest to 1:1 is the most stable.
- \(\ce{^{70}_{33}As}\) is more stable than \(\ce{^{73}_{33}As}\). \(\ce{^{73}_{33}As}\) has an odd number of protons (33) but an even number of neutrons (40). \(\ce{^{70}_{33}As}\) has an odd number of protons (33) and an odd number of neutrons (37). Therefore, since it is more likely that an even(p)-odd(n) exist than an odd(p)-odd(n) exist, \(\ce{^{73}_{33}As}\) is more stable. Also the ratio of neutrons to protons in \(\ce{^{73}_{33}As}\) is 1.21 which is closer to the ideal ratio of 1.5 for \(\mathrm{20\leq Z < 83}\). The ratio of \(\ce{^{70}_{33}As}\) is 1.12.
- \(\ce{^{182}_{74}W}\) is more stable than \(\ce{^{189}_{74}W}\). First, \(\ce{^{182}_{74}W}\) has an even number of protons (74) and an even number of neutrons (182) while \(\ce{^{189}_{74}W}\) has an even number of protons (74) and an odd number of neutrons (115). Simply because of this, \(\ce{^{182}_{74}W}\) would be more stable. By calculating the ratio of neutrons to protons, \(\ce{^{182}_{74}W}\) is again found to be more stable since its ratio is 1.46 and is thus closer to the ideal ratio of 1.5 for \(\mathrm{20\leq Z < 83}\). \(\ce{^{189}_{74}W}\)'s ratio of neutrons to protons is 1.55.
For review on this topic, visit the page "Decay Pathways" .
Q25.42D
Which member of the following pairs of nuclide would you expect most abundant in natural sources. Explain your answer
- Y-89 or Y-91
- Cs -132 or Cs- 130
S25.42D
- Y-89 is more abundant, because it is closer to the average atomic mass of Y, which is 88.91.
- Cs-132 is more abundant, because it is closer to the average atomic mass of Cs, which is 132.9.
Q25.42E
Of the following pairs, explain which of the two atoms is more stable and why?
- \(\ce{^{44}_{22}Ti}\) or \(\ce{^{46}_{22}Ti}\)
- \(\ce{^{34}_{17}Cl}\) or \(\ce{^{35}_{17}Cl}\)
- \(\ce{^{66}_{32}Ge}\) or \(\ce{^{65}_{32}Ge}\)
S25.42E
- \(\ce{^{44}_{22}Ti}\) because a 1:1 proton neutron ratio is associated with stability for elements with \(\mathrm{z<20}\)
- \(\ce{^{35}_{17}Cl}\) because both isotopes have an odd number of protons, but only \(\ce{^{35}_{17}Cl}\) has an even number of neutrons
- \(\ce{^{66}_{32}Ge}\) because an even number of neutrons is associated with stability
Write a Nuclear Equation for the Alpha Decay of 241 95 Am
Source: https://chem.libretexts.org/Bookshelves/General_Chemistry/Exercises:_General_Chemistry/Exercises:_Petrucci_et_al./25:_Nuclear_Chemistry

0 Response to "Write a Nuclear Equation for the Alpha Decay of 241 95 Am"
Post a Comment